1. $y = \frac{1}{x}$ #
The derivative of $y = \frac{1}{x}$ can be computed in the following way.
First, I highly recommend you to watch this clip , where 3blue1brown visualizes this function.
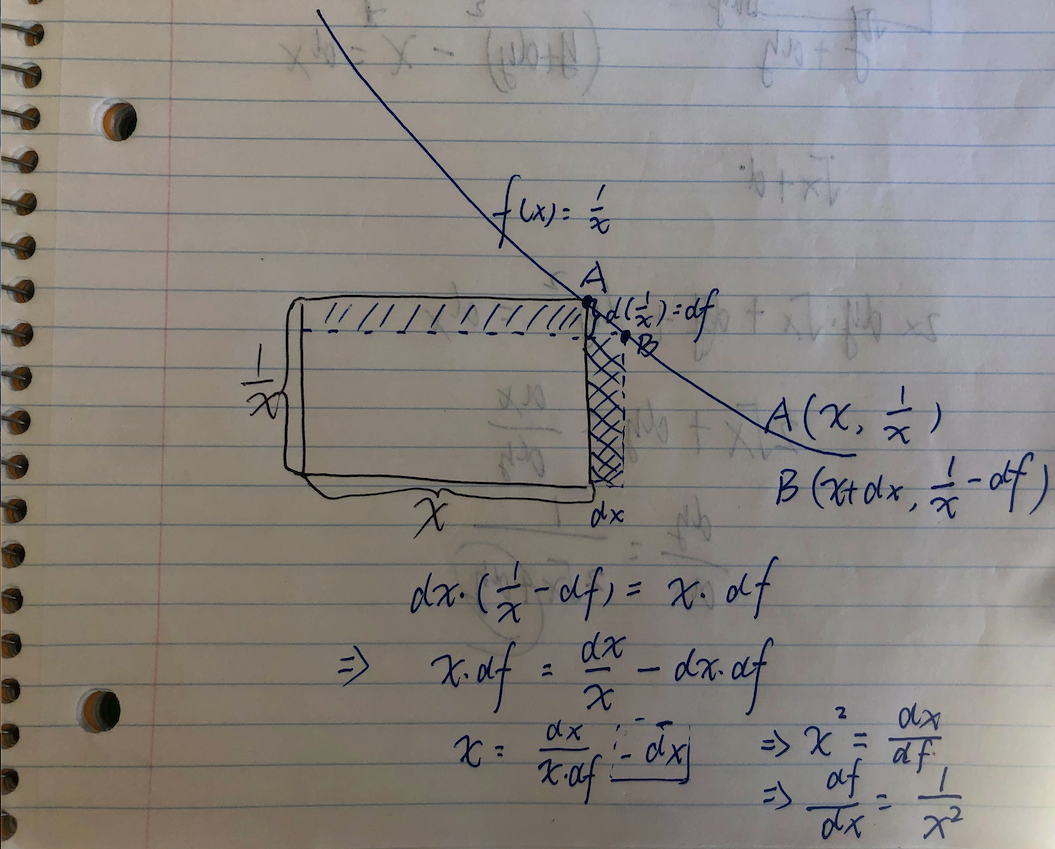
The key part of the proof is that Since A and B are both on the curve of $y = \frac{1}{x}$, the x coordinate times the y coordinate is 1. That is to say: $A_x \cdot A_y = 1$ and $B_x \cdot B_y = 1$. If you know this, then you’ll know that the areas of the two shaded areas are equal. Therefore,
$$dx \cdot (\frac{1}{x} - df) = x \cdot df$$
We have:
$$x\cdot df = \frac{dx}{x} - dx\cdot df$$
So:
$$x = \frac{dx}{x \cdot df} - dx$$
Because $dx$ is extremely small, we can safely ignore it, and therefore, we have:
$$x = \frac{dx}{x \cdot df}$$
Multiply the above equation by $x$ and we have:
$$x^2 = \frac{dx}{df}$$
So we have:
$$\frac{df}{dx} = \frac{1}{x^2} = x^{-2}$$
Because $df$ is negative, the derivative should be negative as well, so:
$$\frac{df}{dx} = - \frac{1}{x^2} = - x^{-2}$$
This method is inspired by F J .
2. $y = \sqrt{x}$ #
It can be proven in two ways.
Intuitive way #
First, let’s use the way suggested by 3blue1brown.
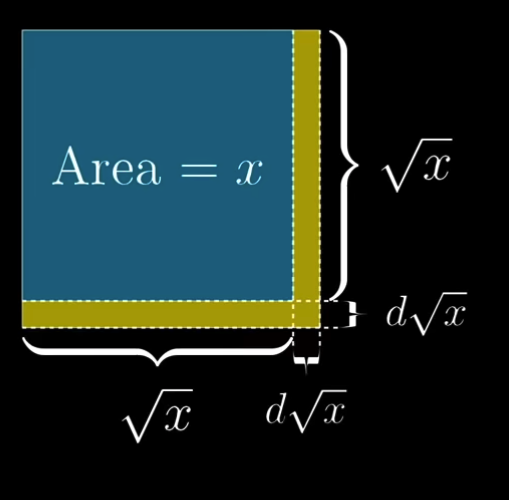
Source: Chapter 3 of Essence of calculus by 3blue1brown
I’ll write $d\sqrt{x}$ as $dy$.
We have
$$dx = 2\sqrt{x}\cdot dy + (dy)^2$$
Divide the equation by $dy$ and we have:
$$2\sqrt{x} + dy = \frac{dx}{dy}$$
Therefore,
$$\frac{dy}{dx} = \frac{1}{2\sqrt{x} + dy}$$
Because $dy$ is approaching zero, we can safely ignore it and we have
$$\frac{dy}{dx} = \frac{1}{2\sqrt{x}} = \frac{1}{2}x^{-\frac{1}{2}}$$
Chain rule #
Let’s say we have $f(x) = \sqrt{x}$, $g(x) = x^2$, and $h(x) = g(f(x)) = (\sqrt{x})^2 = x$.
We have:
\begin{align} h^\prime(x) & = 1 = g^\prime(f(x)) \cdot f^\prime(x)\\ & = 2\cdot f(x) \cdot f^\prime(x) \\ & = 2\sqrt{x} \cdot f^\prime(x) \\ \end{align}
So we have
$$f^\prime(x) = \frac{1}{2\sqrt{x}}$$
The method by chain rule is inspired by Yifan Wei.
#MLLast modified on 2025-06-05 • Suggest an edit of this page